Journal of Statistics Education, V8N2: Yesilcay
Research Project in Statistics: Implications of
a Case Study for the Undergraduate Statistics
Curriculum
Yasar Yesilcay
James Madison University
Journal of Statistics Education v.8, n.2 (2000)
Copyright (c) 2000 by Yasar Yesilcay, all rights
reserved. This text may be freely shared among individuals,
but it may not be republished in any medium without
express written consent from the author and advance
notification of the editor.
Key Words: Data analysis; Data
specialist; Graduation project; Undergraduate curriculum;
Undergraduate research.
Abstract
A Research Project in Statistics is proposed as a
major requirement of undergraduate statistics curricula to
provide hands-on experience to students and equip them with
the tools they will need after graduation. Such a
requirement will train students to solve real-life problems
by choosing a statistical model suitable to a problem,
learning the details of that model, collecting and
analyzing appropriate data, and interpreting the results
obtained. After completing the project, students will have
the ability to learn new techniques on their own, to do a
literature review, and to carry out sample and survey
design, and they will have enhanced their oral and written
reporting skills. The case study reported in this paper
suggests that students tend to learn more by doing such a
project than in any regular coursework. The project is
motivating and gives students a feeling of working in an
almost real-life environment on a real problem. Such
a project incorporates many aspects of the nonmathematical
courses suggested by Higgins
(1999a) and is expected to better prepare students to
meet the needs of potential employers.
1. Introduction
1 Many undergraduate statistics curricula are designed to
train students for graduate work, with many courses in
mathematics, probability, and statistical theory. This is
the impression of Higgins
(1999a, pp. 1, 2): "I think it is safe to say
that most statistics departments began with a central
mission to produce M.S. and Ph.D. statisticians to fill
needs in academia, government, and industry.… The
undergraduate discipline of statistics is a discipline in
search of an identity. Administrators, colleagues,
students, and prospective employers think it is a branch of
mathematics." Even statisticians may think of statistics as a branch of
mathematics: "Statistics is, or should be, about
scientific investigation and how to do it better, but many
statisticians believe it is a branch of mathematics" (Box 1990, quoted in Higgins
1999a, p. 2).
A possible reason for this is that many undergraduate
statistics programs are in mathematics departments.
Another reason might be that most statisticians were
themselves mathematics majors as undergraduates.
2 Undergraduate statistics programs that exist within
mathematics departments incorporate many of the statistics
courses listed in the guidelines considered by the American
Statistical Association (ASA) (Pirie
1986) and suggested by the recommendations of the ASA-MAA Joint Committee on Undergraduate Statistics (Cobb 1992). However, the number of such
courses is limited because of the courses required in
mathematics. This, and various other limitations imposed by
being part of a mathematics department, have led many
statisticians to suggest that statistics programs should be
carried out in independent statistics departments (de Leeuw 1995, Ahmad
1995, Kettenring 1997).
3 These and other observations have led Higgins (1999a, p. 1) to suggest an
undergraduate curriculum that includes nonmathematical
courses for teaching students "things [that] are very
much a part of what a practicing statistician does and what
customers of statistics need." In addition to some of
the traditional courses in statistics and mathematics,
Higgins proposes eight new, nonmathematical courses:
- The Scientific Process,
- Planning and Managing Surveys,
- Planning and Managing Scientific Experiments,
- Statistical Software for Data Management,
- Statistical Graphics,
- Computer Science in Statistics,
- Communicating Statistical Ideas, and
- Management Principles for Statistics.
4 In 1989, aware of the need for the subjects covered in
the nonmathematical courses suggested by Higgins (1999a), the Department of
Mathematics and Statistics at Sultan Qaboos University in
Oman required all senior students to take a
nine-credit-hour, two-semester "course" entitled
Research Project in Statistics. Such a research
project incorporates at least some parts of the above
listed courses, as well as the suggestions for
"partnership in the extended community" and
"interaction with industry and government" of Hogg (1999a, pp. 10, 11). It also
includes an emphasis on data in the undergraduate
statistics curriculum (Cobb 1999, Higgins 1999a). In the next three
sections of this paper, the implementation of the
Research Project in Statistics at Sultan Qaboos
University in Oman and its implications for the
undergraduate statistics curriculum will be explained. The
possibility of merging Higgins’ ideas with the
Research Project in Statistics will be explained in
the last section.
2. Research Project in Statistics at Sultan Qaboos University
5 The Sultanate of Oman is a small country with a
population of about 2.2 million. Although the country has a
long history, in 1970 it had what the Omanis called "a
renaissance" (Azzi 1973). Since
then, every effort has been made by the current ruler,
Sultan Qaboos, to bring the country from the middle
ages to the twentieth century. One area of
major success was education. Before 1970, the country had
only three schools for boys with fewer than a thousand
students. The current educational system includes boys and
girls as well as adults, with more than half a million
students. This is about a quarter of the total population,
with almost equal numbers of boys and girls.
6 In September 1986, Sultan Qaboos University (SQU), the
first university in the country, opened its doors to
students. The curricula in the colleges of science and
engineering take five years. The aim of the first year
courses is primarily to teach the English language and to
make up for any deficiencies in the required background. In
these two colleges and in the College of Medicine, all
courses are taught in English (except the course on Arabic
literature and language and a few elective courses).
Curricula for every department of the university were
prepared by British educators prior to 1986. However, at
the beginning of the academic year 1988-1989, the
university administration decided to have all curricula
based on the "American system."
7 With the needs of the country in mind, the Department
of Mathematics and Computing (later called the Department
of Mathematics and Statistics), revised the contents of the
courses and the requirements for the three majors --
statistics, mathematics, and computer science -- offered by
the department. A departmental curriculum committee
composed of one representative of each discipline and a
convenor worked to set up the curricula for these degrees.
After long discussions in committee meetings and then in
the Department Board, the changes were approved by the
department, college, and the university.
8 The new curriculum in statistics has the usual
mathematics and statistics courses suggested in the 1986
ASA guidelines (Pirie 1986).
However, there is one major difference: students are
expected to carry out a Research Project in
Statistics before they graduate. This is a nine-credit-hour, two-semester "course," in which
every senior student is expected to work on a real-life
problem, as a member of a team of usually three students.
9 The essence of the projects was explained by the
chairman of the department at the time (Eltayeb 1994):
All senior students of the Department of
Mathematics and Statistics at Sultan Qaboos University
carry out scientific projects, which deal with problems of
Mathematics and/or Statistics. This activity is considered
an important ingredient for the training of good graduates.
It is aimed to train the students to design a
mathematical/statistical model for a real problem, analyze
the model, solve it and interpret the results with respect
to the real situation.
Supervision of these
projects is demanding but we believe that it affects the
quality of the graduate. It is known that the graduation
project provides a good opportunity for the students to
solve a real life problem. Despite the difficulties
encountered by the students in this respect (because they
need to use diverse technical material and they must be
able to inter-relate different concepts) they have shown
the highest degree of motivation.
10 Although the content of various courses has been
modified over time, the research course has remained
virtually untouched. Such a project course became so
popular among the students that other departments in the
College of Science introduced similar requirements in their
curricula. Details of the procedure for implementing this
course are given below.
2.1 Preliminary Work
11 Towards the end of every
academic year, the statistics faculty announces a list of
research problems that are relevant to the community. For
this purpose, the upper level administrators in various
government offices, banks, and some large private companies
are contacted during the year to find problems that may be
formulated as a research project in statistics. Although
one could use the research topics listed in the works of Scott (1976), Anderson and Loynes (1987), or Conrad (1989), these references were
hard to find in the library of a new university with
limited books. Each project has two statisticians as
project co-supervisors.
12 A few
days after the research topics are announced, the students,
who are likely to graduate in two or three semesters,
report their order of preference for each project. They
also give the names of at least two students with whom they
would like to work. The project coordinator matches the
projects with teams, trying to satisfy as many students as
possible. The list of projects chosen for the next academic
year and the members of the team for each project are thus
determined and announced before the end of the year.
13 During the summer months,
students may join the relevant agency for an
"apprenticeship in statistics." Although summer
work is strictly voluntary, many students choose to do it.
This gives them an opportunity to see the agency, to get to
know the people who work there, and to learn about the
research problem. It also gives them opportunities to be
known by the agency for future employment. A significant
number of graduates have found their first jobs in this
way.
2.2 Activities in the Fall Semester
14 Students register for a three-credit component of the
"course" during the fall semester. During this
semester, and to some extent during the voluntary summer
semester, they define the problem and try to find some
suitable statistical techniques that may be used. Teams
meet with their project supervisors weekly and give
presentations on what they have done and learned (on their
own) during the week. Supervisors probe and then clarify
the vague points in these presentations. Students learn the
details of the techniques they need, with minimum support
from the supervisors. (See the next section.) By the end of
the semester, the literature review, model selection, and
preparations for data collection are completed.
15 Around the middle and at the
end of the fall semester, each student submits an
independent progress report. These reports explain what the
student understands from the problem definition,
statistical techniques, and data collection, as well as any
other aspects of the project on which they have worked.
These reports help the project supervisors to assess the
contributions of each student. This is needed in the final
grading of students. The progress reports also help all
team members to contribute to the first draft of the
written report for the project.
2.3 Activities in the Spring Semester
16 Students register for a six-credit component of the
"course" during the spring semester. Data
collection, analysis, interpretation, and reporting are all
done in this term. Some teams carry out the data collection
and/or other activities during the semester break. Students
have a division of labor at this stage, but they are still
responsible for the whole project. As in the fall semester,
weekly meetings with the supervisors continue.
17 About a week before the end of
the spring semester, there is a "Project Presentation
Day" where all the projects of the department are
presented to the public. Although these oral presentations
are not graded, students get very excited because this is
their first public appearance in front of a large crowd.
There is further reason for excitement: their families,
friends, and professors, as well as potential employers,
are all in the audience. Furthermore, the event gets media
coverage by the radio, television, and some newspapers of
Oman. At the end of the oral presentations, students answer
questions asked by anyone in the audience. Their professors
make sure that there are enough questions for everyone.
18 Starting with the progress
reports submitted during the fall semester, teams draft
final written reports on their projects. The drafts are
revised and re-revised during the semester. This is the
most time consuming stage of the project for both students
and supervisors. Two weeks before the oral examination
day, these reports are submitted to all members of the
examination committee. Committee members comment on these
reports and suggest corrections. These comments and
corrections must be incorporated into the final reports. The
final reports are then photocopied, bound, and submitted to
the department, college, and the supervisors.
19 The last one or two days of the
final examination period is allocated for the oral
examination of the projects. A committee of at least four
professors carries out the oral examination: two project
co-supervisors, the project coordinator (for the whole
department), and a statistics professor who was not
involved in the supervision of the project. Students are
called in one at a time and examined on the complete
report. Each committee member asks one or two questions.
After the last question, the student leaves the room. Each
member of the committee assigns a numerical grade for the
oral examination based on responses to all questions asked.
These are given to the project coordinator. Then the next
student in the team is called in. The oral examination of
each student takes about 20 minutes.
20 Each student gets a single grade for the nine-credit
two-semester course. This is calculated as the weighted
average of the following four components:
- Grade given by each member of the examination
committee for the written report. (Every member of
the team gets the same grade.)
- Grade given by each member of the examination
committee for the oral examination. (Each student may
get a different grade.)
- Grades given by the two co-supervisors for the
contribution of each student to the project. (Each
student may get a different grade.)
- Grade given by the project coordinator for the
whole project, to reflect the "success" of
each project relative to others. (Every member of the
team gets the same grade.)
The project coordinator gets all of the above grades and
calculates a weighted average of the grades for each
student. Then members of all examination committees meet
to assign letter grades to the numerical averages, without
identifying students or projects.
21 The Research Project in Statistics covers a
wide range of topics and gives students an experience that
is not possible to give through regular courses. A partial
list of projects carried out between 1990 and 1996 is given
in Table 1 to give an idea of the
range of subjects covered. (See the Appendix for summaries of these
projects.)
Table 1. A Partial List of Project Titles From
the Research Project in Statistics at Sultan Qaboos University
"Factors that Influence the Success of Students in
Sultan Qaboos University"
"Statistical Modeling in Inventory Decisions and an Application"
"National Diabetes Survey in Oman: Regional Prevalence Rates
and their Relation to Risk Factors"
"A Queuing Model for Customer Service at a Local Bank"
"An Educational Advancement Markov Model for the Secondary
School System in Oman"
"Some Statistical Analyses of the Results of Benchmark Tests
on Computational Hardware"
"Progress and Prospects of Oman Through Statistics: A
Tribute for the 25th National Day"
"Machine Repair Problem: An Analysis of Simulated Data"
|
3. Some Attractive Features of the Research Project in Statistics at Sultan Qaboos University
22 Incorporating a research project within a course is
not a new idea. It is used in many statistics courses,
including introductory statistics courses (for example, see
Smith 1998 and Anderson and Sungur 1999). It is
also one of the recommendations of the ASA-MAA Joint
Committee on Undergraduate Statistics (Cobb 1992). However, a
"course" only for statistical research seems to
be new, although it is used in other disciplines. (See, for
example, the Journal of Undergraduate Research
of Harvard University or Morehead Electronic Journal
of Applications in Mathematics of Morehead State
University.) The following are some of the attractive
features of the SQU course that make the Research
Project in Statistics so valuable.
23 Real-Life Problems. Most of the
topics covered in the projects were obtained from
government agencies, banks, or large private sector
companies. They dealt with real problems that these
agencies needed to study or were in the process of
studying. Some topics were suggested by project supervisors
to these agencies and were carried out if these agencies
showed any interest and promised cooperation.
24 Problem Definition. Even in some simple
exercises in elementary statistics courses, students have
difficulties in identifying or defining the problem
correctly (Gardner and Hudson
1999). This step is the most difficult one in the
project as well. Some simplifying assumptions are
necessarily made in defining the problem. However, students
and their supervisors ensure that these assumptions will
not make the defined problem unrealistic or its solution
unusable.
25 Model Selection. Although the students are
guided in the selection of an appropriate model, the final
decision is always theirs. They are asked to justify their
choice of model both in the written report and in the oral
examination. Students must be prepared to answer questions
on why they did not choose other models that might seem to
be appropriate.
26 Independent Study. The project supervisors will
help students identify a broad group of statistical
techniques, such as multivariate statistics, regression
analysis, time series analysis, or categorical analysis,
where one or more applicable methods might be found.
Students may not have already studied these subjects.
Students are then asked to study these techniques on their
own, initially skipping the formulae and derivations,
trying to answer the following questions for each
technique:
- Under which conditions can one use this technique?
- What are the implicit or explicit assumptions and requirements?
- What kind of questions can be answered when this technique is used?
- What type(s) of data are needed?
- Can one collect such data?
- Is there any software available to carry out this analysis?
- Is there any need for programming to transform and/or analyze the data?
- How is the output interpreted?
27 Each student in the team studies one or more of the
techniques that seem to be relevant. They present the
techniques to their teammates and supervisors during the
meetings of the first two or three weeks, answering the
above questions and any other questions their supervisors
or teammates may ask. Once all these techniques are
reviewed, the team starts discussing which one(s) may be
used for the problem at hand and reach a decision. After
this, all formulae, their derivations, and relevant
literature are reviewed. In this way, students learn not
only the techniques they need for that specific project,
but they also get an idea about other related or similar
techniques, with emphasis on similarities and differences
among them.
28 The independent learning process is aimed to be as
close to real-life learning as possible. The supervisors
try to simulate what students are likely to face after
graduation: there is a problem to be solved. A review of
the literature reveals that other people faced with the
same or similar problems have used certain techniques. Some
of these techniques are covered, to some extent, in the
undergraduate curriculum, and hence students have some
familiarity with them. Other researchers have used
techniques that are new to the students. They look at the
references for these techniques, try to find as many as
they can, and read and understand them, keeping in mind
that they may be questioned about them during the
presentations (and the oral examination). If some point is
not clear, they try to consult an expert (their supervisors
and/or other faculty in the department).
29 To what extent is this learning process successful? It
certainly depends on the level of difficulty of the
techniques involved, as well as the ability of the students.
In general, if the technique involved is one that is
covered in one or more of the courses taken, students get
further insight into the subject and get a real
understanding of it. On the other hand, if it is a new
subject not covered in any course, the depth of
understanding of the subject could be quite limited.
However, even there, students learn the existence of such a
technique, have some understanding of how it is used, what
software is available to use it, the type of data used, and
how the output is interpreted. Compared to the alternative
of no such exposure, the experience gained is definitely
worth the effort.
30 Teamwork and Leadership. It is natural that
when students graduate, they will work as a member of a
team. Furthermore, in future years, they are likely to
become leaders of teams. Supervisors encourage each student
to be a leader in some part of the project, thus letting
their leadership characteristics come to the surface. As
team leaders, students must organize the material, assign
duties to their teammates, finalize that aspect of the
project, and write the relevant part of the final report.
As members of the team, they carry out the activities
assigned by the team leader and contribute to the success
of the team. Thus, they get their first "on the
job" training in teamwork and leadership when they
work on their projects. This turns out to be an invaluable
experience that cannot be obtained elsewhere.
31 Survey Design. Most projects are formulated to
require some data collection activity on a relatively small
scale. In this activity, students see the need for a
detailed plan covering all phases from the clarification of
survey objectives to the final report. They are made
aware that survey design is not the same thing as sample
design. In some projects, the agency has already collected
the data. Reports in such cases include the details of the
survey plan used by the agency, along with a critique of
the survey design.
32 Sample Design and Data Collection. This is the
stage where students "get their hands dirty" with
data collection. They see the problems related to working
with real data. They do their own sample design,
construction of frame(s), sample selection, locating the
sampled units, and the collection of data. When needed, the
team may get help in data collection from their friends on
other teams. This is encouraged because it exposes
students to other problems of data collection, such as rejection by sample members or sample members that are
not at home, and teaches students how to handle
them.
33 Data Analysis. This is usually the easiest part
of the project. Students use some commercial software
package for the analysis. In this way, they learn to use
such software if they were not exposed to
it in their previous courses. They may also write some
small, special purpose programs for some data management
activities such as consistency checks, data editing, or
transformation.
34 Data Management. When large datasets are used
for projects, students need to learn how to manage such
data. Even with small datasets, the process of data entry
and the search for inconsistent data, outliers, and other
errors, as well as their correction or deletion, give
students some knowledge of data management.
35 Interpretation. Students interpret the output
obtained from some software package or from the special
purpose programs they write in terms of the problem
specified. This seems to be a difficult step, especially
when the output obtained does not give a direct answer to
the problem specified. This step teaches students to keep
in mind the survey objectives at every step of the
research.
36 Report Writing. This is the hardest part of the
project, because the native language of the students is not
English. However, the challenge is to organize
ideas and express them in such a way that someone
with limited or no knowledge of statistics can still
understand most of the report. This step teaches the
students to express technical ideas in non-technical terms
for non-statisticians. The project coordinator is usually a
mathematics professor with limited knowledge of statistics,
and one of his tasks is to check the reports and make sure
that they are understandable by non-statisticians.
37 Oral Presentation. Although the oral
presentation is not graded, students spend a lot of time
preparing for it. Repeated rehearsals and preparation of
back-up plans in case something goes wrong teach them to be
prepared before such a presentation. Usually a PowerPoint
presentation is used, but students must be ready to use
overhead transparencies, and they may add slides, videos,
or special purpose tools to their presentations. Each
student presents a part of the project.
38 Public Speaking. Making presentations to an
audience is a difficult task for new graduates in many
cultures. It becomes especially hard in a culture in which
it is considered "bad manners" for a young person
to speak in front of elders or for a female to speak in
front of males. Yet, they need to do it if they are going
to be professionals: they need to talk with their
colleagues and bosses, individually or in groups, large and
small. Oral presentations to teammates during the year, as
well as a presentation to a much larger group at the end of
the year, provide valuable experience that cannot be
taught by coursework alone.
39 Employment Opportunities. As stated in the
previous section, many potential employers of the
graduates, especially those who "own" the project
problems, are invited to the oral presentation. This is a
great opportunity for students to show their knowledge and
for potential employers to seek the best among the
graduates. It is also a good chance to promote the
department and the university. Thus, the university gives
full support to such activities.
4. Some Challenges in Implementing the Research
Project in Statistics at Sultan Qaboos
University
40 Although the Research Project in Statistics has
many valuable aspects, the Department of Mathematics and
Statistics at SQU has faced a number of challenges, and
many hours have been spent in committee and department
meetings to resolve them.
41 One major difficulty with
such a course is the pressure it exerts on the resources of
the department and the university, especially in terms of
the number of project supervisors (statistics professors)
and the amount of technical support needed. Part of the
problem is that the Department of Mathematics and
Statistics at SQU assigns two co-supervisors, rather than
one, to each project. This is done as a precaution, to
avoid any possible interruption in the flow of research.
Some consider this over-cautious. However, even if one
faculty member is assigned to each project, this may still
require three to seven faculty members in a small
department that has only ten to 15 seniors (since each team
has two or three students). This becomes a serious problem
in small departments, especially when the number of
students majoring in statistics is large.
42 The allocation
of workload for faculty members who supervise such projects
and for the project coordinator is difficult. Some
faculty members believe that supervision and coordination
are light work, and hence that the coordinator and each
supervisor should be assigned one or at most two
credit-hours of workload per semester for each project with
which they are involved. Others believe that supervision is
hard and time-consuming work and should carry the full nine
credit-hours of workload per year. Some believe that
supervision should carry at least three credit-hours load
per semester. A few faculty members state that they prefer
to teach three regular three-credit-hour courses rather
than supervise a project. (In Oman, supervisors spend quite
a lot of time editing the project reports to have them in
proper English. This may be mainly due to English being the
second language of the students. It may be different in
other universities.) In departments of mathematics where
there is a large discrepancy between the numbers of
students majoring in mathematics and in statistics, the
assignment of the workload may cause friction between
mathematicians and statisticians. This is especially true
when there are no students majoring in mathematics.
43 Grading has been a challenging task at
SQU. One difficulty relates to assigning different
grades to students on the same team. This problem is
addressed by grading various components of the project
activities and then using a weighted average of all grades
assigned by the examiners and the project coordinator for
each student. However, it has been difficult to reach
consensus on the weights assigned to each component.
Another source of friction results from different examiners
using different yardsticks in their grading of the same or
similar projects. Although this also occurs in other
courses with many sections taught by many faculty, it
becomes a more serious problem when the grades are for the
same course. One of the duties of the (departmental)
project coordinator, who is the chairman of every
examination committee, is to "smooth out" the
differences in the grading process of different projects.
Although this puts a lot of pressure on the coordinator, it
seems to work reasonably well at SQU.
44 Although the problem of drop-outs was
not faced at SQU, one must think about what to do in case a
team member cannot (or will not) continue until the end of
the project. Such a problem may arise for family or health
reasons. Alternatively, a student may not wish to work on a
project during the spring semester because of poor
performance (in other courses or on the project) during the
fall semester. Another possibility is that a student is
dismissed or has to leave the university for a certain
period for disciplinary reasons. A possible solution is to
redefine the problem to reduce the work required of the
remaining students.
45 In spite of all the difficulties involved in
administering the Research Project in Statistics, it
has become a permanent part of all undergraduate degree
programs in the College of Science at SQU because of the
many benefits observed of having such a component of the
curricula.
5. Merging the Research Project in Statistics With Nonmathematical Courses
46 In this information age, "the collection,
management, and analysis of data are essential to today's
society. The things that are at the core of the discipline
of statistics are things that society demands.… These
things are very much a part of what a practicing
statistician does and what customers of statistics
need" (Higgins 1999a, p. 1).
Furthermore, "there is potentially a big demand for
the data specialist" (Higgins
1999a, p. 5). To meet these demands, we must revise
and extend undergraduate statistics programs. Iman (1994) and Mittal
(1994) point to the urgency of the problems faced by
statistics departments, as does Hogg
(1999a, p. 14): "We find that many other areas,
certainly computer science and several in engineering and
business colleges, have taken much of the action away from
us, and we cannot afford to lose anymore if we do not want
the statistics profession to atrophy."
47 The Research Project in Statistics carried out
in the Department of Mathematics and Statistics at SQU lets
students implement some of the ideas in the courses
proposed by Higgins. If the core four-year sequence of new
and traditional undergraduate courses suggested by Higgins (1999a, p. 5) is
supplemented by a Research Project in Statistics,
the result will be much better than either initiative
alone. Such a merger will create graduates who will meet
the needs of employers. It will also provide a stronger
background in applied statistics for those who want to
continue graduate studies.
48 Incorporating a Research Project in Statistics
into an undergraduate statistics curriculum will naturally
require deleting two or three other courses. Because some
of the objectives of the nonmathematical courses suggested
by Higgins (1999a) will be met (to
a large extent) by the Research Project in
Statistics, the new courses could be limited to five or
six of the eight listed. The decision on which courses to
delete depends on a number of considerations, such as what
is available on the campus and the interests of the
statisticians in the department.
49 Furthermore, some courses, such as a course in
Communicating Statistical Ideas or one on
Planning and Managing Surveys would be difficult to
organize and teach on a "stand alone" basis. This
becomes especially difficult when one considers the
limitations on textbooks written for these subjects.
However, one can cover these subjects within the context of
a Research Project in Statistics.
50 Similarly, a course on Management Principles for
Statistics may resemble a course in Management
Principles as taught in a college of business. Students
may be advised to take such a course as an elective from
that college to satisfy some other requirement. (For
example, they may take it as a "non-technical
elective" or a "general education
elective.") Although the emphasis of such a course may
be different from what Higgins
(1999a) had in mind, students would get at least the
basic ideas of management and learn the terminology to
communicate with people in this field. The same suggestion
can be made for a course on The Scientific Process.
This should be a required course for all science students
(and an elective for other students). Finally, one may
merge the courses on Statistical Software for Data
Management with Computer Science in Statistics
or with Statistical Graphics. As stated before, the
final decision on which courses to keep depends on the
resources available and the interests of the statistics
faculty, as well as other factors.
51 Articles and replies by Higgins (1999a, 1999b)
and Hogg (1999a, 1999b), as well as discussions by
Newton, Cobb,
Bryce, and Groeneveld, all of which appeared
in The American Statistician (February 1999),
have started an excellent discussion on "Undergraduate
Statistics Education: What Should Change?" These
articles motivated the present paper. The expected benefits
of proposed changes in undergraduate statistics education
can be amplified by adding an applied statistics research
requirement to the undergraduate statistics curriculum. In
this way, students will not only get the information needed
to become successful professionals, but will also have a
chance to implement many of these ideas before graduation.
The outcome of such a program will be a very strong cohort
of young statisticians who are ready to meet the needs of
society. They will be data specialists who can do even some
sophisticated data analyses.
Acknowledgments
The author would like to thank his colleagues and
students at Sultan Qaboos University who have shaped the
project course. He would like to express his
sincere thanks to the referee and especially to the
Associate Editor and to Professor Stanislaw Swierczkowski
for their invaluable comments and suggestions.
Appendix: Brief Summaries of the Projects Listed in
Table 1
Listed below are the titles and abstracts (as written by
the students) of the projects co-supervised by the author
between 1990 (when the project requirement was introduced) and 1996
(when the author left the university). The list does not
include projects that were supervised by other faculty
members in the department.
"Factors that Influence the Success of Students in
Sultan Qaboos University"
A systematic random sample of 500 students for all colleges
of the university was selected using the university
registration files. These students were given a short
questionnaire designed to investigate the factors that
influence their academic success. Various statistical
techniques were employed to find out these factors.
"Statistical Modeling in Inventory Decisions and an
Application"
Inventory modeling is considered as one of the techniques
in Operations Research. This project consisted of mainly
three parts. The first part was a relatively extensive
literature survey. This was followed by some original
mathematical derivations for a stochastic "lot size,
reorder point model" under several assumptions for the
lead time distribution. The final part consisted of two
case studies, one being a simulated problem to give the
students first-hand experience and insight. The second case
study analyzed the actual inventory policy of an Omani
company involved in importing foodstuff. The project was an
integrated work with mathematical, statistical,
computational, communicational and managerial skills being
used extensively to produce the final report.
"National Diabetes Survey in Oman: Regional Prevalence
Rates and their Relation to Risk Factors"
The National Diabetes Survey was designed and data were
collected by the Ministry of Health in 1990. This project
aimed at analyzing the data for the survey. An extensive
literature survey was conducted and summarized in the final
report. Descriptive as well as advanced statistical
techniques were employed to estimate the prevalence of
Diabetes on a regional and national basis and to relate it
to the presence of some risk factors such as hypertension,
obesity and several blood chemical measurements. The
findings, conclusions and suggestions were summarized in
the report.
"A Queuing Model for Customer Service at a Local
Bank"
In this project, a queuing model is used to analyze the
customer-service relation in the Sultan Qaboos University
branch of Al-Bank Al-Ahli Al-Omani. It is observed that,
although a high percentage of customers are satisfied with
the duration of service, a significant percentage found it
to be long or too long. Estimated parameters of the model
indicated that the mean waiting time could be reduced
drastically by increasing the number of computer terminals
from one to two. Although the cost of this change is
minimal, it will increase customer satisfaction by
decreasing the length of the long queues during the busy
hours. The report includes other suggestions for increasing
customer satisfaction.
"An Educational Advancement Markov Model for the
Secondary School System in Oman"
A statistical model called educational advancement model
is used as a tool for predicting the number of students in
each grade of the secondary schools in Oman, as well as the
number of graduates, for the academic years 1992/1993 to
2000/2001. The model is tested using simulations, which
indicated that the assumptions of the model are justified,
and that the predictions are expected to be within 7% of
the true values. The predicted numbers indicate that the
total number of students in the secondary school system are
expected to increase from about 40 thousand in 1992/1993 to
almost 100 thousand in 2000/2001. The number of graduates is
predicted to almost triple from 9409 to 26451. These imply
that the work loads in the secondary school system as well
as the institutes of higher education will be almost triple
at the turn of the century. The report ends with some
suggestions for further research in this field.
"Some Statistical Analyses of the Results of Benchmark
Tests on Computational Hardware"
Computers are important devices in our lives. People face
some difficulties in choosing personal computers from the
many different brands in the market. In this project, some
tools were developed for use by buyers to help them find
the "best PC" for their needs. These tools were
developed using multivariate analyses of benchmark test
results on computational hardware. It was possible to group
173 different brands of PCs into three
"equivalent" classes. The functions obtained were
able to discriminate between these classes with a very
small probability of misclassification. The performance of
some 386 type PCs were found to be as good as the 486 type
PCs. However, most of the 386 type performs worse than the
486 type.
"Progress and Prospects of Oman Through Statistics: A
Tribute for the 25th National Day"
The improvements in the lives of Omani people have been
analyzed and projected to the year 2000 using some
statistical forecasting techniques. The aspects analyzed
are education, health, employment, transportation and
communications. The analyses indicate that there are
remarkable improvements in the variables studied and the
trends are likely to continue in the near future, with more
students, teachers, classrooms, doctors, nurses, hospital
beds and telephone lines. There will be more vehicles on
longer roads and many telephone calls (both business and
private) will be made. The number of Omanis employed in the
Public sector is predicted to increase at a decreasing
rate, reaching a ceiling around the turn of the century;
the number of non-Omanis employed in Oman is expected to
decrease. All of these indicate that the living conditions
of the Omanis have been improving and further improvements
are expected.
"Machine Repair Problem: An Analysis of Simulated
Data"
Most countries of the world are living in the industrial
era and many are moving towards the information age. One of
the main characteristics of these two periods is that
almost all goods and services are produced by machines.
Although a lot of research and development work is carried
out to increase the reliability of these machines, they
still fail at some stage of production and must be
repaired, thus creating the machine repair problem. In a
company with N machines, subject to a failure rate
of 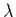 , and some given cost
parameters, the management may call r (
, and some given cost
parameters, the management may call r ( 1) repairmen as soon as
m (
1) repairmen as soon as
m ( 1) machines
fail. The project looks for the optimum repair policy in
terms of r and m. To this end, we developed
and simulated a machine repair model and analyzed the
simulation data to find the optimum values of r and
m for different
1) machines
fail. The project looks for the optimum repair policy in
terms of r and m. To this end, we developed
and simulated a machine repair model and analyzed the
simulation data to find the optimum values of r and
m for different 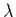 , that yield the minimum average annual
cost.
, that yield the minimum average annual
cost.
References
Ahmad, I. A. (1995),
"Statistics Needs Academic Independence,"
Amstat News, 224, 14.
Anderson, C. W., and Loynes, R. M.
(1987), The Teaching of Practical
Statistics, New York: Wiley.
Anderson, J. E., and Sungur, E. A.
(1999), "Community Service Statistics
Projects," The American Statistician,
53, 132-136.
Azzi, R. (1973), "Oman, Land of
Frankincense and Oil," National
Geographic, 143(2), 205-230.
Box, G. (1990), "Commentary on
Communications Between Statisticians and Engineers/Physical
Scientists," Technometrics, 32,
251-252.
Bryce, G. R. (1999),
"Discussion," The American
Statistician, 53, 21-23.
Cobb, G. W. (1992), "Teaching
Statistics," in Heeding the Call for Change:
Suggestions for Curricular Action, ed. L. A. Steen,
Washington, DC: Mathematical Association of America, pp.
3-43.
----- (1999),
"Discussion," The American
Statistician, 53, 15-21.
Conrad, S. (1989),
Assignments in Applied Statistics, Wiley: New
York.
de Leeuw, J. (1995), "UCLA
Launches Statistics Department," Amstat
News, 224, 14.
Eltayeb, I. A. (1994), 1994
Annual Report of the Department of Mathematics and
Statistics, Departmental Archives, Department of
Mathematics and Statistics, Sultan Qaboos University,
Oman.
Gardner, P. L., and Hudson, I.
(1999),
"University Students' Ability to Apply Statistical
Procedures," Journal of Statistics
Education [Online], 7(1).
(https://www.amstat.org/secure/v7n1/gardner.cfm)
Groeneveld, R. A. (1999),
"Discussion," The American
Statistician, 53, 23-25.
Higgins, J. J. (1999a),
"Nonmathematical Statistics: A New Direction for the
Undergraduate Discipline," The American
Statistician, 53, 1-6.
----- (1999b), "Reply,"
The American Statistician, 54, 27-28.
Hogg, R. V. (1999a), "Let's Use
CQI in Our Statistics Programs," The American
Statistician, 54, 7-14.
----- (1999b), "Reply,"
The American Statistician, 54, 26-27.
Iman, R. L. (1994), "Statistics
Departments Under Siege," Amstat News,
212, 6-7.
Kettenring, J. (1997),
"The Birth, Life and Death of Statistics
Departments," Amstat News, 245, 9.
Mittal, Y. (1994), "Continuing
the Debate on ‘Statistics Under Siege’: We Must
Promote Vigorous Growth in the Statistics
Departments," Amstat News, 213,
21-22.
Newton, H. J. (1999),
"Discussion," The American
Statistician, 53, 15-16.
Pirie, W. R. (1986), "The
Changing State of Undergraduate Statistics Education:
Guidelines for Majors," in Proceedings of the
Section on Statistical Education, American Statistical
Association, pp. 43-45.
Scott, J. F. (1976), "Practical
Projects in the Teaching of Statistics at
Universities," The Statistician, 25,
95-108.
Smith, G. (1998), "Learning
Statistics by Doing Statistics," Journal of
Statistics Education [Online], 6(3).
(https://www.amstat.org/v6n3/smith.html)
Yasar Yesilcay
James Madison University
MSC 7803
Department of Mathematics and Statistics
Harrisonburg, VA 22807
Yesilcay@math.jmu.edu
JSE Homepage | Subscription
Information | Current Issue |
JSE Archive
(1993-1998) | Data Archive | Index | Search JSE
| JSE
Information Service | Editorial Board |
Information for
Authors | Contact JSE | ASA
Publications
 1) repairmen as soon as
m (
1) repairmen as soon as
m ( 1) machines
fail. The project looks for the optimum repair policy in
terms of r and m. To this end, we developed
and simulated a machine repair model and analyzed the
simulation data to find the optimum values of r and
m for different
1) machines
fail. The project looks for the optimum repair policy in
terms of r and m. To this end, we developed
and simulated a machine repair model and analyzed the
simulation data to find the optimum values of r and
m for different